
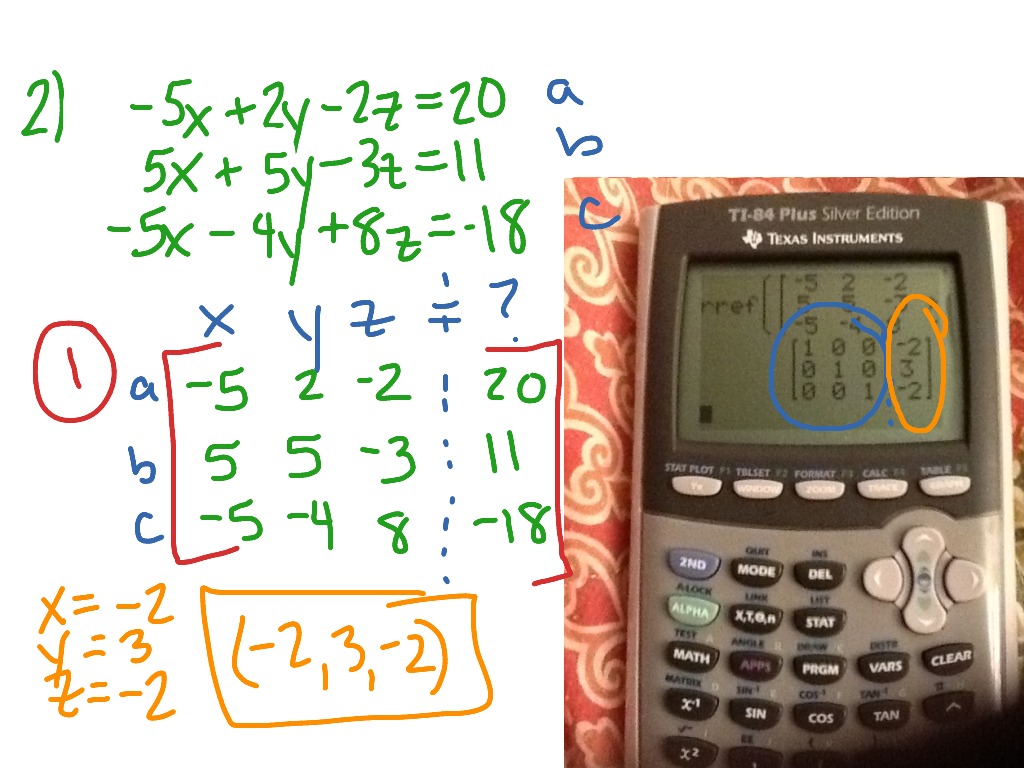
When you plug this in to the second equation, you get: Since V is likely easier to work with in the second equation (the substituted term is likely easier to multiply by 1.5 than by 1.25), you can leverage the first equation to get P in terms of V: This problem sets up nicely for using the Substitution Method for systems of equations.
Then for the money, the total revenue will be a combination of how much was made from selling vegetarian slices ($1.25 times the number of vegetarian) and how much was made from selling pepperoni ($1.50 times the number of pepperoni). If you use V to represent the number of vegetarian slices and P to represent the number of pepperoni slices, you can express the total number of slices as: This problem provides you with the opportunity to set up two equations: the total number of slices of pizza, and the total amount of money. That means that R = 48, but remember in Systems of Equations problems to always check that your algebra has answered the specific question, as a problem with two variables allows you to mistakenly solve for the wrong one! The question asks you about Spot's weight, so you can subtract 9 from 48 to see that Spot weighs 39 pounds. If you structure it that way, you can stack the equations and sum them: The equation with subtraction allows you to go straight into the Elimination Method as you have a + S in the first equation and a -S in the second, so that may be your preferred choice. Another way is to see that Rover weighs more, and the difference is 9, meaning that R - S = 9. One is to say that "Rover's weight is 9 pounds more than Spot's weight" and then use the fact that "is" means "equals" so that the words translate directly to the equation: R = 9 + S.
System of equations solver show work how to#
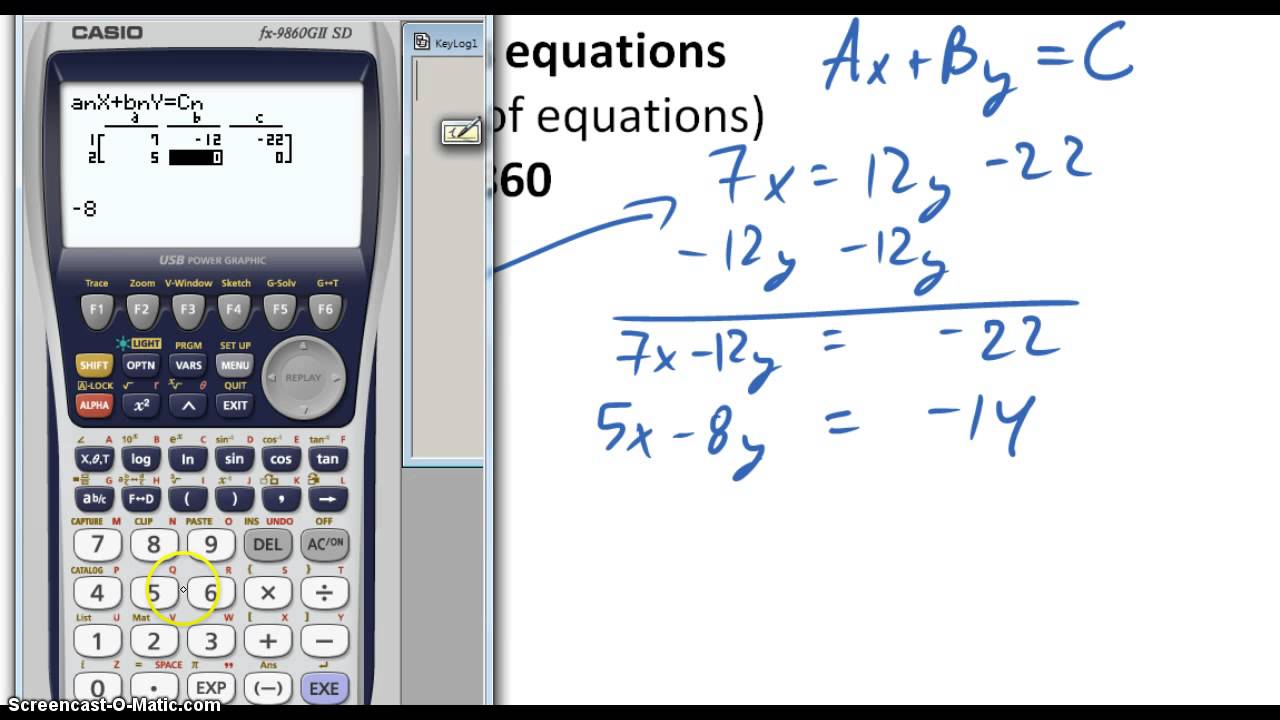
Then if Rover weighs 9 pounds more than Spot, you have a few choices for how to express that. R + S = 87 (in which R = Rover's weight and S = Spot's weight) If the two dogs weigh a total of 87 pounds, you can express that as: Often on the SAT, these Systems problems will start by giving you a combined total as this one does. This problem provides you with two equations, allowing you to use Systems of Equations logic to solve once you've constructed the equations.

System of equations solver show work plus#
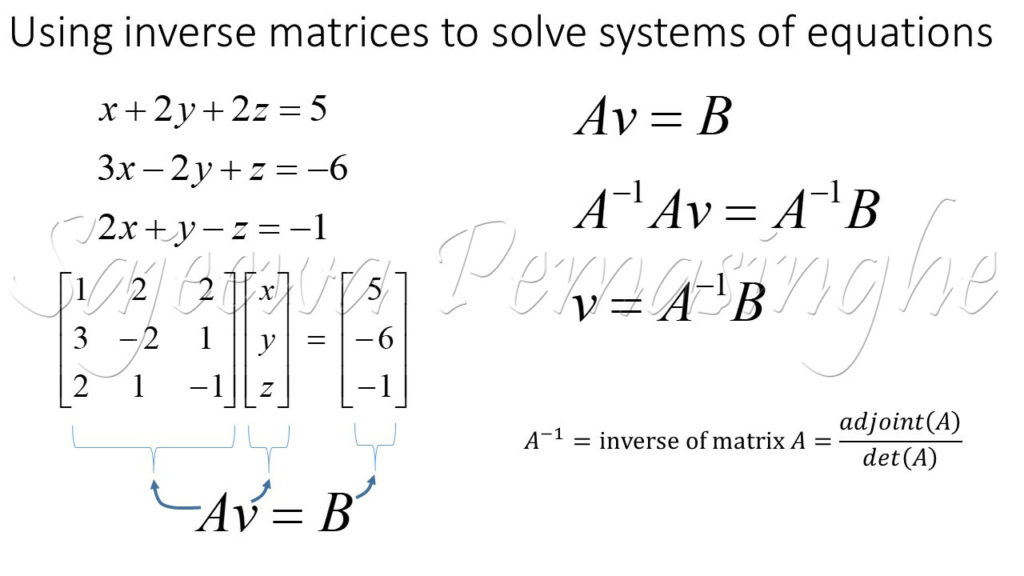
Then check your work: does Blin's total of $150,000 plus Alex's total of $90,000 arrive at the sum we know to be $240,000? It does, so you can confidently choose $150,000. The question asks you about Blin's total, not Alex's, so you need to add $60,000 to get to Blin's total of $150,000. Of course, at this point you need to check whether you solved for the proper variable. This structure sets up well for Substitution you already have B in terms of A, so you can plug in the second equation for the first: For the relationship equation, you can say that Blin's total is $60,000 more than Alex's, which translates to B = 60,000 + A. The sum equation is generally straightforward you can express that as B + A = 240,000. Here that's Blin raised $60,000 more than Alex did. You're given the sum of two entities - here that's Blin's Total + Alex's Total = $240,000 - and a relationship between those two entities. This problem provides you with a classic SAT Systems of Equations setup.


 0 kommentar(er)
0 kommentar(er)
